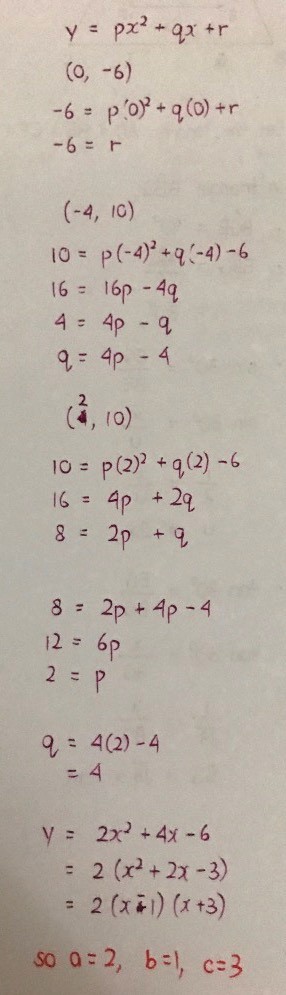Eric Nicholas K's answer to MM's Secondary 4 E Maths Singapore question.
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
This should be easier to understand
Date Posted:
4 years ago
Yes second one is easy
To add,
There are three formats to consider.
1. y = ax² + bx + c
2. y = a (x + b) (x + c)
3. y = a (x + h)^2 + k
Which is the best approach to use, or are there times where one approach is preferred?
---------------------------------------------
1. y = ax² + bx + c
This approach is preferred when the y-intercept of the graph is provided and two other points on the curve are provided, as is the case in this question.
If (0, -6) is a point on the curve, then c = -6.
We use the other two points (-4, 10) and (2, 10) to form two simuls in two unknowns and solve.
---------------------------------------------
2. y = a (x + b) (x + c)
This approach is preferred when the TWO x-intercepts are provided and one more point is provided.
Suppose the x-intercepts are -3 and 1. Then we have y = a (x + 3) (x - 1). We use the third coordinate to obtain the value of a.
---------------------------------------------
3. y = a (x + h)^2 + k
This approach is preferred when the turning point of the curve is provided and one other point is provided.
If the turning point of the curve is (-1, -8), then by default, h = 1 and k = -8.
Now we have one unknown a left, and we just need one other coordinate (0, -6) to obtain the value of a.
There are three formats to consider.
1. y = ax² + bx + c
2. y = a (x + b) (x + c)
3. y = a (x + h)^2 + k
Which is the best approach to use, or are there times where one approach is preferred?
---------------------------------------------
1. y = ax² + bx + c
This approach is preferred when the y-intercept of the graph is provided and two other points on the curve are provided, as is the case in this question.
If (0, -6) is a point on the curve, then c = -6.
We use the other two points (-4, 10) and (2, 10) to form two simuls in two unknowns and solve.
---------------------------------------------
2. y = a (x + b) (x + c)
This approach is preferred when the TWO x-intercepts are provided and one more point is provided.
Suppose the x-intercepts are -3 and 1. Then we have y = a (x + 3) (x - 1). We use the third coordinate to obtain the value of a.
---------------------------------------------
3. y = a (x + h)^2 + k
This approach is preferred when the turning point of the curve is provided and one other point is provided.
If the turning point of the curve is (-1, -8), then by default, h = 1 and k = -8.
Now we have one unknown a left, and we just need one other coordinate (0, -6) to obtain the value of a.
Take note of these as it simplifies your workings greatly.
Thank you for going the extra mile to help me out. Thank you so mucb