Daniel Poon's answer to chloe's Secondary 4 A Maths Singapore question.
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
This question seems challenging at first, but once you understand what the condition really means, you will be able to solve it very quickly.
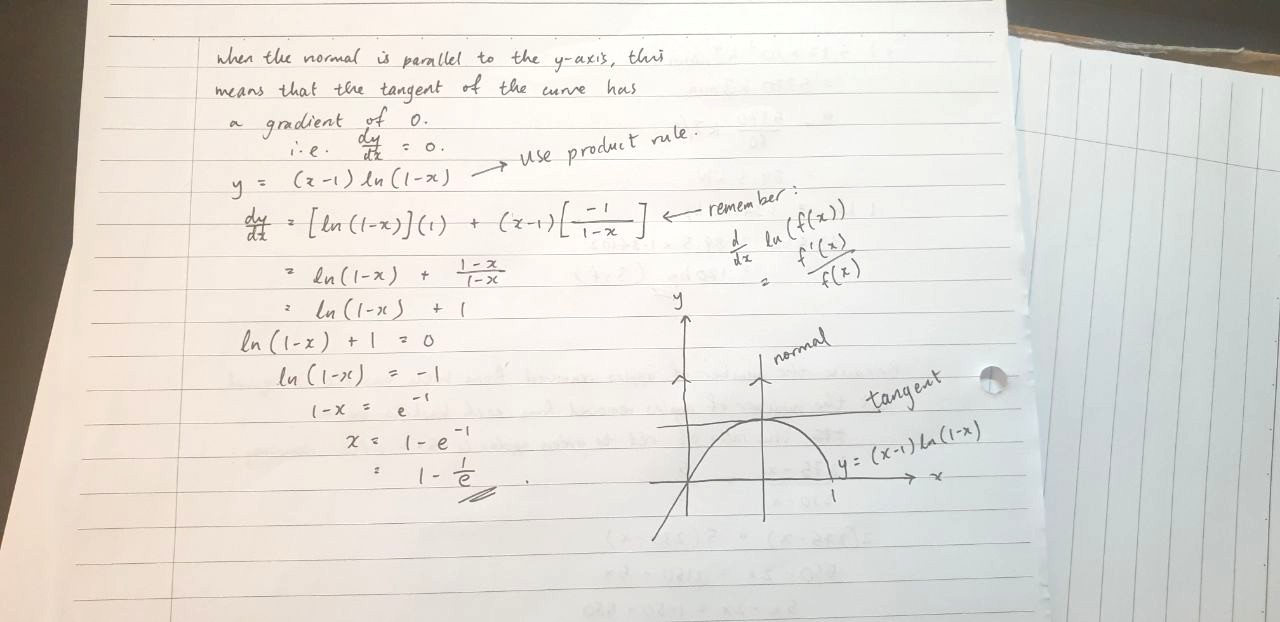
As a visualisation, I have sketched out the positive x and y axes and the graph of y = (x - 1) ln (1 - x). The graph is an increasing function for x < 0, so this is the only point where the normal to the graph is parallel to the y-axis. Do not worry about what the graph looks like because you are not required by the A-Math syllabus to know the appearance or shape of this graph. As you can see, when the normal to the graph is parallel to the y-axis, this means that the tangent to the graph is parallel to the x-axis (i.e. dy/dx = 0).
Find dy/dx using the product rule. Recall the differentiation of logarithmic functions as well. Solve for x and leave the answer in exact terms.
As a visualisation, I have sketched out the positive x and y axes and the graph of y = (x - 1) ln (1 - x). The graph is an increasing function for x < 0, so this is the only point where the normal to the graph is parallel to the y-axis. Do not worry about what the graph looks like because you are not required by the A-Math syllabus to know the appearance or shape of this graph. As you can see, when the normal to the graph is parallel to the y-axis, this means that the tangent to the graph is parallel to the x-axis (i.e. dy/dx = 0).
Find dy/dx using the product rule. Recall the differentiation of logarithmic functions as well. Solve for x and leave the answer in exact terms.
Date Posted:
2 years ago
360 Tutoring Program