Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.

Question
junior college 1 | H1 Maths
2 Answers Below
Anyone can contribute an answer, even non-tutors.

Need help for part(iii) and (iv) urgent!!
P (both are different colour)
= P(1st red, 2nd pink)
+ P(1st pink, 2nd red)
+ P(1st red, 2nd blue)
+ P(1st blue, 2nd red)
+ P(1st pink, 2nd blue)
+ P(1st blue, 2nd pink)
= 10/25 x 8/24
+ 8/25 x 10/24
+ 10/25 x 7/24
+ 7/25 x 10/24
+ 8/25 x 7/24
+ 7/25 x 8/24
= 2(10 x 8 + 10 x 7 + 8 x 7)/(25 x 24)
= 412/600
= 103/150
P(both different colour)
= 1 - P(both same colour)
= 1 - P(both red) - P(both blue) - P(both pink)
= 1 - 10/25 x 9/24 - 7/25 x 6/24 - 8/25 x 7/24
= 1 - (10x9 + 7x6 + 8x7)/(25x24)
= 1 - 188/600
= 1 - 94/300
= 206/300
= 103/150
See 2 Answers

They stated P(both same colour)
= 2/5 x 3/8 + 8/25 x 5/12 + 7/25 x 5/12
① Only the first product of fractions is correct.
2/5 x 3/8 was actually simplified from 10/25 x 9/24.
The 10/25 referred to 10 reds out of all 25 socks for the 1st sock taken.
The 9/24 referred to 9 reds out of 24 remaining socks for the 2nd sock taken.
So this = P(both are red) ✓
BUT,
② 8/25 x 5/12 is wrong.
8/25 x 5/12 was simplified from 8/25 x 10/24.
The 8/25 referred to 8 pinks out of all 25 socks for the 1st sock taken.
The 10/24 referred to 10 reds out of 24 remaining socks for the 2nd sock taken
This probability = P(1st pink,2nd red). But the correct one should have been P(both pink)
Likewise,
③ 7/25 x 5/12 is also wrong.
7/25 x 5/12 was simplified from 7/25 x 10/24.
The 7/25 referred to 7 blues out of all 25 socks for the 1st sock taken.
The 10/24 referred to 10 reds out of 24 remaining socks for the 2nd sock taken
This probability = P(1st blue,2nd red) , not P(both blue) when it should have been.




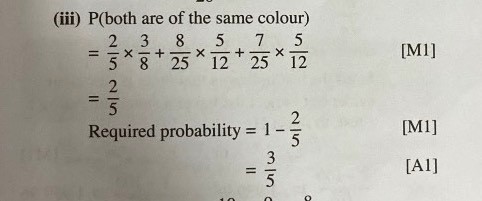

P(at least 2 red socks out of 3 now)
= P(all 3 red)
+ P(2 red, 1 other colour)
= P(all 3 red)
+ P(1st red, 2nd not red, 3rd red)
+ P(1st red, 2nd red, 3rd not red)
+ P(1st not red, 2nd red, 3rd red)
= 10/25 x 9/24 x 8/23
+ 10/25 x 15/24 x 9/23
+ 10/25 x 9/24 x 15/23
+ 15/25 x 10/24 x 9/23
= (10x9x8 + 3x10x15x9)/(25 x 24 x 23)
= 4770/13800
= 477/1380
= 159/460
Shouldn’t it be
P(all 3 red)
+ P(1st red x 2nd red x 3rd pink)
+ P(1st red x 2nd red x 3rd blue)
+ P(1st pink x 2nd red x 3rd red)
+ P(1st blue x 2nd red x 3rd red)
P(1st red, 2nd blue, 3rd red)
P(1st red, 2nd pink, 3rd red)
I choose to combine them together and do the various orders of 2 red, 1 non-red
(Non-red already covers both pink and blue)
You can try to do for the separate case and you should still get 159/460