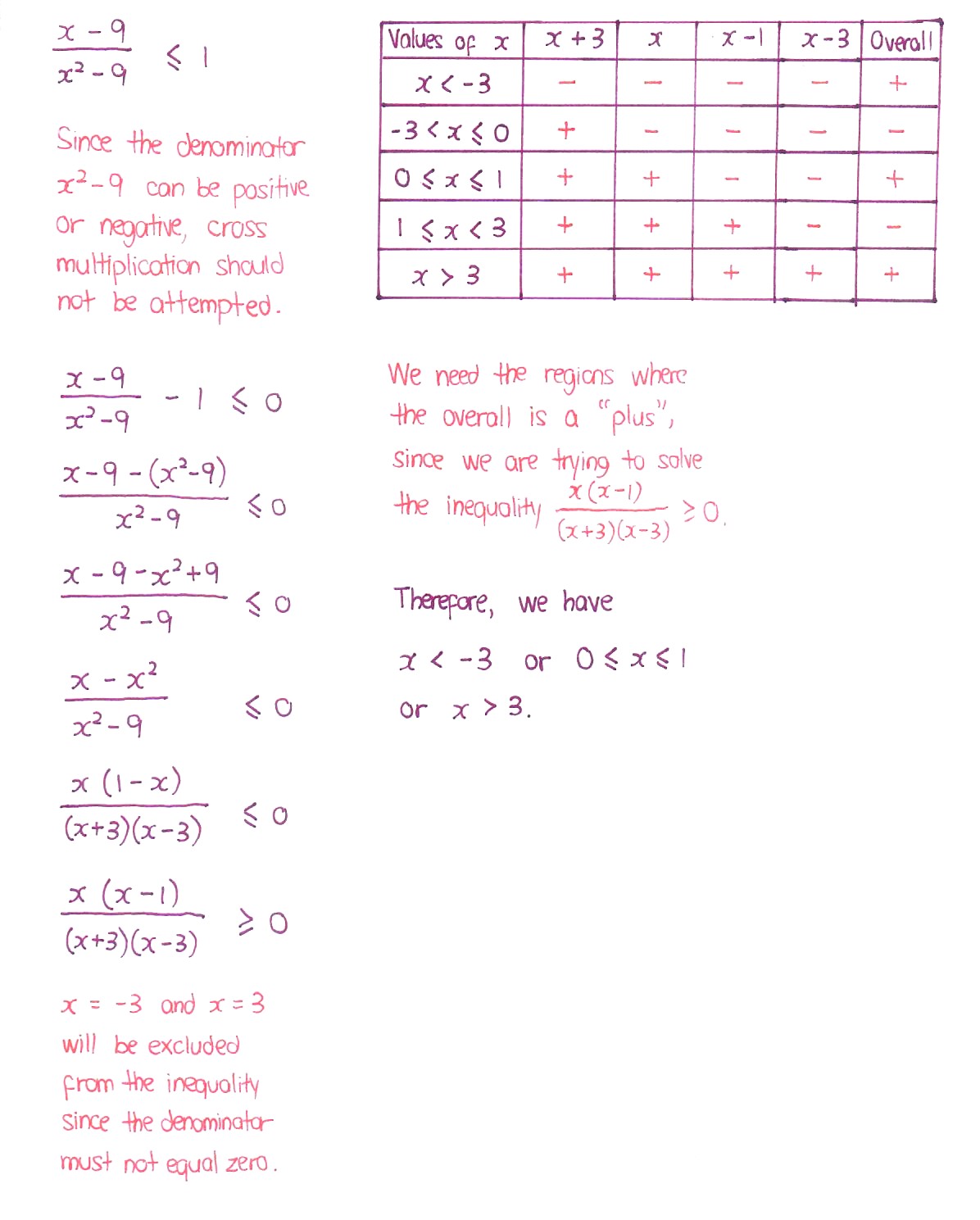
Eric Nicholas K's answer to Candice's Junior College 1 H2 Maths Singapore question.
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
Good evening Candice! Here are my workings for the first inequality.
The remaining are substitutions such as x --> |x|, x --> -x and x --> 1/x.
The remaining are substitutions such as x --> |x|, x --> -x and x --> 1/x.
Date Posted:
4 years ago
better to label x = 1 and x = 0 in a separate category in the table. The current one suggests that there are two possible signs for x for x = 0, and also for x-1 when x = 1
Or,
Change the following :
-3 < x ≤ 0 to -3 < x < 0
0 ≤ x ≤ 1 to 0 < x < 1
1 ≤ x < 3 to 1 < x < 3
Change the following :
-3 < x ≤ 0 to -3 < x < 0
0 ≤ x ≤ 1 to 0 < x < 1
1 ≤ x < 3 to 1 < x < 3
True, but the equals case is more or less deducible from there.
Candice, what J meant is that for the x = 0 and the x = 1 case, the entire expression equal zero. As such, we can classify these two cases as a separate case for the above (just like how I listed out the rejected cases as separate from the other regions).
In effect, we have "purely less than" or "purely more than" signages.
You can modify the cases to become
1. x < -3
2. x = -3, which is the rejected case
3. -3 < x < 0
4. x = 0, which forms part of the equals case
5. 0 < x < 1
6. x = 1, which forms part of the equals case
7. 1 < x < 3
8. x = 3, which is the rejected case
9. x > 3
We can then pick out the relevant regions from there. J's explanations are more accurate than mine, since technically I should not include the equals case there.
Candice, what J meant is that for the x = 0 and the x = 1 case, the entire expression equal zero. As such, we can classify these two cases as a separate case for the above (just like how I listed out the rejected cases as separate from the other regions).
In effect, we have "purely less than" or "purely more than" signages.
You can modify the cases to become
1. x < -3
2. x = -3, which is the rejected case
3. -3 < x < 0
4. x = 0, which forms part of the equals case
5. 0 < x < 1
6. x = 1, which forms part of the equals case
7. 1 < x < 3
8. x = 3, which is the rejected case
9. x > 3
We can then pick out the relevant regions from there. J's explanations are more accurate than mine, since technically I should not include the equals case there.
My concern is more of the loss of marks in presentation due to inaccuracy in the labelling, should the student copy the working verbatim