Chow Tien Wei's answer to Us8s's Junior College 1 H2 Maths Singapore question.
done
2 Upvotes
clear 0 Downvotes

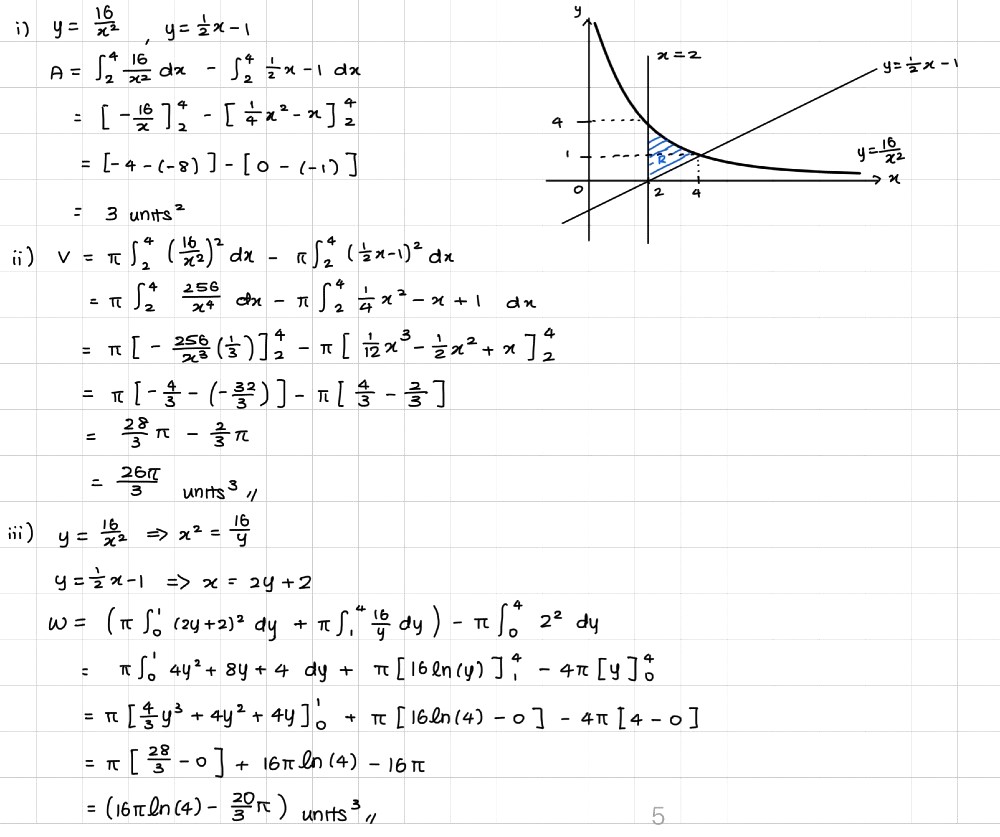
It’s good to try and sketch out the graph if possible to help you to visualise the areas and volumes required. You will also be able to obtain the required limits for the integration.
Part (i): find the resultant area by subtracting the area under the graph for y=16/x^2 with the area for y = (1/2)x -1.
Part (ii): try and visualise the volume of rotation. You will realise that the volume you need is the volume obtained by revolving y = 16/x^2 around the x-axis minus the volume of a cone.
Part (iii): obtain the equations of the two lines in terms of y since you will be revolving around the y-axis. The volume required is the volume obtained by revolving both lines around the y-axis minus the volume of a cylinder.
Part (i): find the resultant area by subtracting the area under the graph for y=16/x^2 with the area for y = (1/2)x -1.
Part (ii): try and visualise the volume of rotation. You will realise that the volume you need is the volume obtained by revolving y = 16/x^2 around the x-axis minus the volume of a cone.
Part (iii): obtain the equations of the two lines in terms of y since you will be revolving around the y-axis. The volume required is the volume obtained by revolving both lines around the y-axis minus the volume of a cylinder.
Date Posted:
2 years ago
Thank u!