Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.
Question
junior college 2 | H2 Maths
3 Answers Below
Anyone can contribute an answer, even non-tutors.
hello can anyone help me with these 2 qns ? Thank u !!
Date Posted: 3 years ago
Views: 682
See 3 Answers
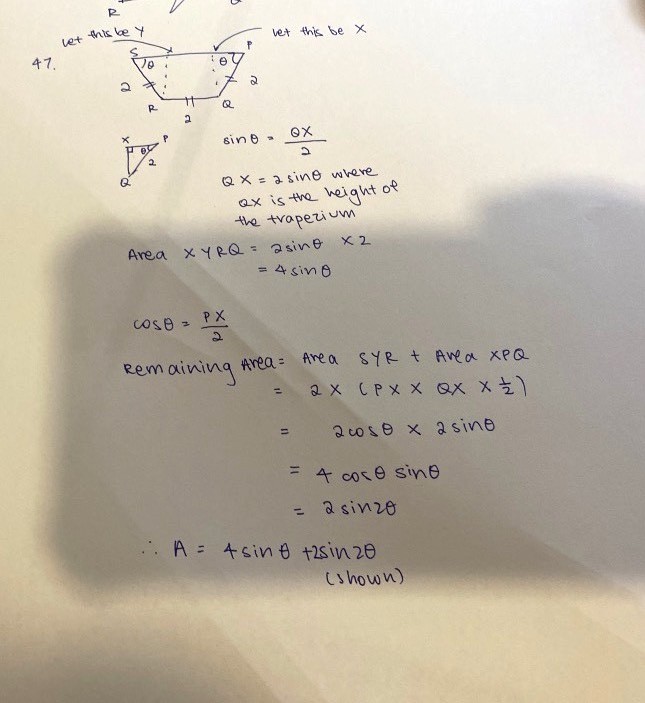
47. This type of trapezium is known as a trisosceles or trilateral trapezium. It has 3 equal sides, two equal base angles as well as two equal top angles.
Divide the trapezium into 3 parts, comprising of :
①A rectangle with one pair of sides 2cm long.
②Two congruent right-angled triangles flanking it, each with hypotenuse of 2cm.
Height of each triangle = breadth of the rectangle = 2 sinθ cm (because using trigo, sin θ = opp/hyp = height/2cm)
Base of each triangle = 2 cosθ cm (using trigo as well)
Area of trapezium = area of rectangle + area of two triangles
= (2 cm)(2 sinθ cm) + 2(½)(2 cosθ cm)(2 sinθ cm)
= 4 sinθ cm² + 4 sinθ cosθ cm²
= 4 sinθ cm² + 2 sin2θ cm²
= (4 + sin θ 2 sin 2θ) cm²
(Shown)
The rest in comments section
Divide the trapezium into 3 parts, comprising of :
①A rectangle with one pair of sides 2cm long.
②Two congruent right-angled triangles flanking it, each with hypotenuse of 2cm.
Height of each triangle = breadth of the rectangle = 2 sinθ cm (because using trigo, sin θ = opp/hyp = height/2cm)
Base of each triangle = 2 cosθ cm (using trigo as well)
Area of trapezium = area of rectangle + area of two triangles
= (2 cm)(2 sinθ cm) + 2(½)(2 cosθ cm)(2 sinθ cm)
= 4 sinθ cm² + 4 sinθ cosθ cm²
= 4 sinθ cm² + 2 sin2θ cm²
= (4 + sin θ 2 sin 2θ) cm²
(Shown)
The rest in comments section
A = 4 sinθ + 2 sin2θ
dA/dθ = 4 cosθ + 2(2)cos 2θ
= 4 cosθ + 4 cos2θ
dA/dt = dA/dθ × dθ/dt
= (4 cosθ + 4 cos2θ)(0.5)
= 2cos π/6 + 2cos 2π/6
= 2(√3 / 2) + 2 (½)
= √3 + 1
dA/dθ = 4 cosθ + 2(2)cos 2θ
= 4 cosθ + 4 cos2θ
dA/dt = dA/dθ × dθ/dt
= (4 cosθ + 4 cos2θ)(0.5)
= 2cos π/6 + 2cos 2π/6
= 2(√3 / 2) + 2 (½)
= √3 + 1
dA/dθ = 4 cosθ + 4 cos2θ
When A has a stationary value, dA/dθ = 0
4 cosθ + 4 cos2θ = 0
cosθ + cos2θ = 0
cosθ + 2cos²θ - 1= 0
(2cosθ - 1)(cosθ + 1) = 0
2cosθ = 1 or cosθ = -1
cosθ = ½ or θ = π,3π,5π,...(N.A as θ is acute)
θ = π/3 (only choose the acute angle)
From here, either do the first derivative or second derivative test.
Second derivative test :
d²A/dθ² = -4 sinθ + 4 (-2sin2θ)
= -4 sinθ - 8 sin2θ
When θ = π/3,
d²A/dθ² = -4 sin π/3 - 8 sin 2π/3
= -4(√3/2) - 8(√3/2)
= -2√3 - 4√3
= -6√3 < 0
(Maximum point)
When A has a stationary value, dA/dθ = 0
4 cosθ + 4 cos2θ = 0
cosθ + cos2θ = 0
cosθ + 2cos²θ - 1= 0
(2cosθ - 1)(cosθ + 1) = 0
2cosθ = 1 or cosθ = -1
cosθ = ½ or θ = π,3π,5π,...(N.A as θ is acute)
θ = π/3 (only choose the acute angle)
From here, either do the first derivative or second derivative test.
Second derivative test :
d²A/dθ² = -4 sinθ + 4 (-2sin2θ)
= -4 sinθ - 8 sin2θ
When θ = π/3,
d²A/dθ² = -4 sin π/3 - 8 sin 2π/3
= -4(√3/2) - 8(√3/2)
= -2√3 - 4√3
= -6√3 < 0
(Maximum point)
48.
x = 4t³, y = 3t⁴
So dx/dt = 12t² , dy/dt = 12t³
Next, dy/dx × dx/dt = dy/dt
dy/dx × 12t² = 12t³
dy/dx = 12t³/12t² = t
So, gradient of tangent to curve C = t
Gradient of normal to C = -1/t
Equation of normal can be written as: y = mx + c
Sub y = 3t⁴ , x = 4t³ and m = -1/t,
3t⁴ = (-1/t)(4t³) + c
3t⁴ = -4t² + c
c = 3t⁴ + 4t²
So equation of normal is : y = -x/t + 3t⁴ + 4t²
x/t + y = 4t² + 3t⁴
x + ty = 4t³ + 3t⁵
(Shown)
x = 4t³, y = 3t⁴
So dx/dt = 12t² , dy/dt = 12t³
Next, dy/dx × dx/dt = dy/dt
dy/dx × 12t² = 12t³
dy/dx = 12t³/12t² = t
So, gradient of tangent to curve C = t
Gradient of normal to C = -1/t
Equation of normal can be written as: y = mx + c
Sub y = 3t⁴ , x = 4t³ and m = -1/t,
3t⁴ = (-1/t)(4t³) + c
3t⁴ = -4t² + c
c = 3t⁴ + 4t²
So equation of normal is : y = -x/t + 3t⁴ + 4t²
x/t + y = 4t² + 3t⁴
x + ty = 4t³ + 3t⁵
(Shown)
48 continued :
Since the normal at t = 2 cuts the curve C again at t = p,
then t = p satisfies the equation of the normal, since that point on C lies on the normal.
Sub t = 2 into equation of normal,
x + 2y = 4(2³) + 3(2⁵)
x + 2y = 32 + 96
x + 2y = 128
Since t = p satisfies the equation, sub t = p, x = 4t³, y = 3t⁴,
4p³ + 2(3p⁴) = 128
6p³ + 4p³- 128 = 0
3p⁴ + 2p³ - 64 = 0
(Shown)
Since the normal at t = 2 cuts the curve C again at t = p,
then t = p satisfies the equation of the normal, since that point on C lies on the normal.
Sub t = 2 into equation of normal,
x + 2y = 4(2³) + 3(2⁵)
x + 2y = 32 + 96
x + 2y = 128
Since t = p satisfies the equation, sub t = p, x = 4t³, y = 3t⁴,
4p³ + 2(3p⁴) = 128
6p³ + 4p³- 128 = 0
3p⁴ + 2p³ - 64 = 0
(Shown)
Thank u !
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
this is the "show" part, doing the next part now! lmk if u need further clarification
Date Posted:
3 years ago
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
a & b
Date Posted:
3 years ago
360 Tutoring Program