Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.

Question
junior college 1 | H2 Maths
One Answer Below
Anyone can contribute an answer, even non-tutors.

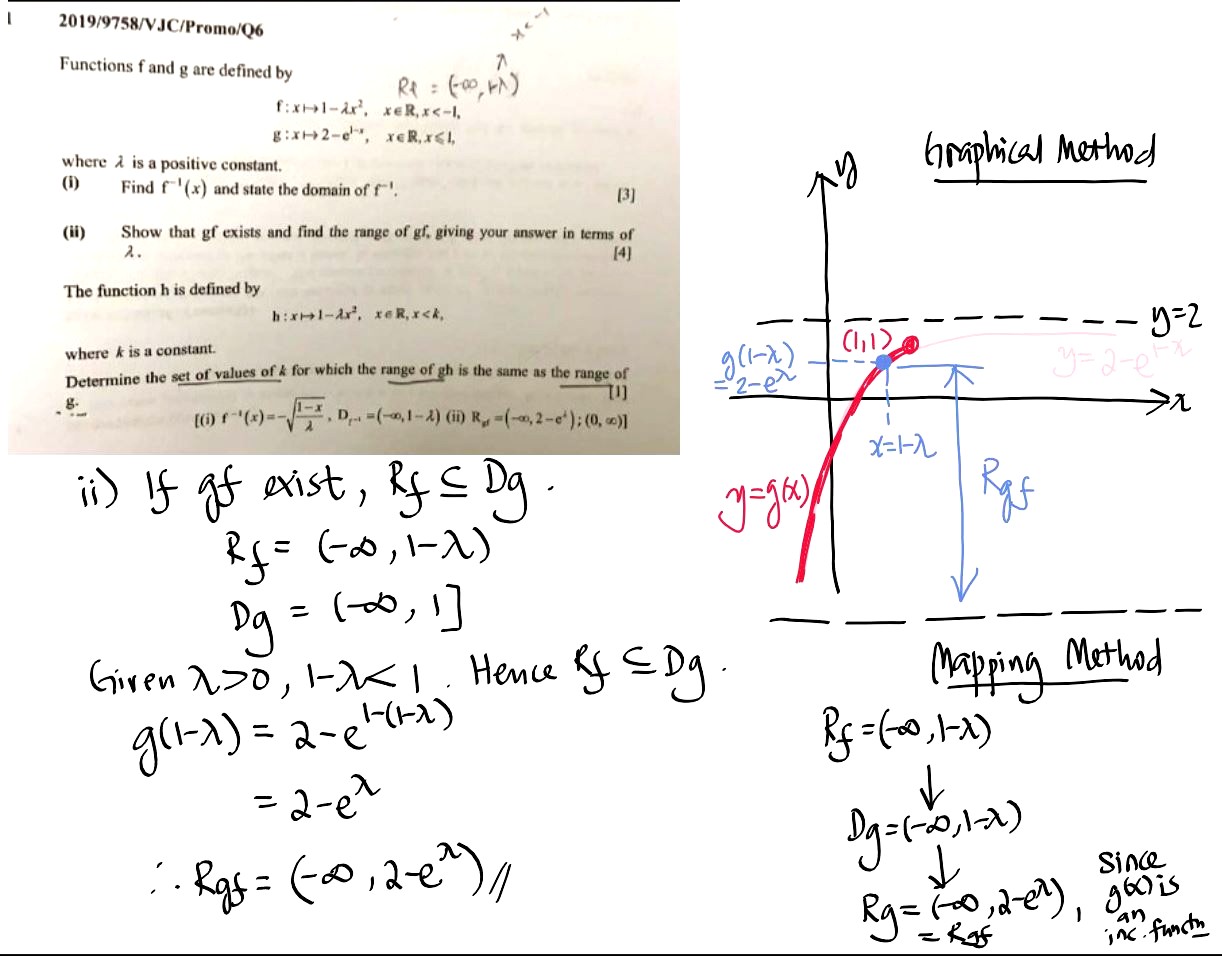
helloo can anyone help me with part (ii) thank you
Rf ⊂ Dg
Dg = (-∞,1]
f(x) = 1 - λx² where x < -1
This is the equation of a quadratic curve which opens downwards. It is symmetric about the y-axis, with the maximum at f(x) = 1 when x = 0.
But since x < -1, we find f(x) when x = -1
f(1) = 1 - λ(1²)
= 1 - λ
So Rf = (-∞, 1 - λ)
Since λ is a positive constant, 1 - λ < 1
So Rf ⊂ Dg and therefore gf exists.
gf(x) = 2 - e^(1 - (1 - λx²))
= 2 - e^(λx²)
Since λ is positive, and x² > 0 for x < -1 ,the exponent is getting bigger as x decreases.
So e^(λx²) strictly increases when x decreases from -1. This implies 2 - e^(λx²) strictly decreases as x decreases.
When x = -1,
gf(-1) = 2 - e^(λ(1²))
= 2 - e^λ
So for x < -1, gf(x) < 2 - e^λ
As x tends to -∞, so does 2 - e^(λx²) since it is strictly decreasing.
Therefore,
Rgf = (-∞, 2 - e^λ)
For Rgh = Rg, the range of h should be exactly the same as the domain of g.
This is because we are substituting the values of h(x) as the x values in g(x) for the composite function.
i.e gh(x) = g(X) , where X = h(x)
So Rh = Dg = (-∞,1]
Since h(x) = 1 - λx², this means that 1 - λx² ≤ 1
λx² ≥ 0
Since λ > 0,
x² ≥ 0
|x|≥ 0
This means x can take any real value, with
i.e x ∈ R, Dh = (-∞,∞)
Since x < k, k cannot be equal or smaller than 0, as this results in 0 being excluded from the domain.
Excluding 0 means x² = 0 is excluded. Then h(0) = 1 - λ(0)² = 1 is also excluded. This would not fulfill the range above.
But as long as k is bigger than 0, every value of x below it can get us to|x| ≥ 0, and will include x = 0
Eg. If x < 0.001 includes x = 0, x = -1 , x = - 100000, x = -0.001 etc.
And |0| = 0, |-1|= 1,|-1000| = 100000, |-0.001| = 0.001
These already make x² ≥ 0 so we need not include positive x values of x ≥ 0.001 in the domain.
So k > 0
Range of k is (0,∞)
g(x) = 2 - e^(1 - x) and Dg = (-∞,1]
1 - x ≥ 0 for x ≤ 1, and increases as x decreases
So e^(1 - x) strictly increases as x decreases from 1. This implies that 2 - e^(1 - x) decreases.
When x = 1, g(1)
= 2 - e^0
= 2 - 1
= 1
As x tends to -∞, so does 2 - e^(1 - x)
So Rg = (-∞,1]
Now h has the same expression as f. They differ in their domain.
So gh(x) also = 2 - e^(λx²)
Since we want Rgh = Rg,
2 - e^(λx²) ≤ 1
e^(λx²) ≥ 1
λx² ≥ 0
x² ≥ 0 since λ is positive. No change of sign is needed.
|x|≥ 0
Apply the same reasoning as before.
See 1 Answer