Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.
Question
junior college 1 | H2 Maths
2 Answers Below
Anyone can contribute an answer, even non-tutors.
hello pls help me with these questions? Thank u!!
Date Posted: 4 years ago
Views: 336
See 2 Answers
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
Q7
For part two, observe that gradient is 0 when t = 1 and gradient = infinity when t is too positive small.
The thing is, the denominator t * e^t is already a small fixed number of e when t = 1, and it gets even smaller when t is lowered to decimals, whereas the numerator "increases in magnitude" from 0 to 1 as t goes from 1 to 9 (the negative sign is for downsloping purposes only).
With the magnitude of the numerator increasing to 1 and the denominator decreasing to 0, it is why the tangent becomes steeper and steeper as t goes to zero.
For part two, observe that gradient is 0 when t = 1 and gradient = infinity when t is too positive small.
The thing is, the denominator t * e^t is already a small fixed number of e when t = 1, and it gets even smaller when t is lowered to decimals, whereas the numerator "increases in magnitude" from 0 to 1 as t goes from 1 to 9 (the negative sign is for downsloping purposes only).
With the magnitude of the numerator increasing to 1 and the denominator decreasing to 0, it is why the tangent becomes steeper and steeper as t goes to zero.
Date Posted:
4 years ago
Thank u!!
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
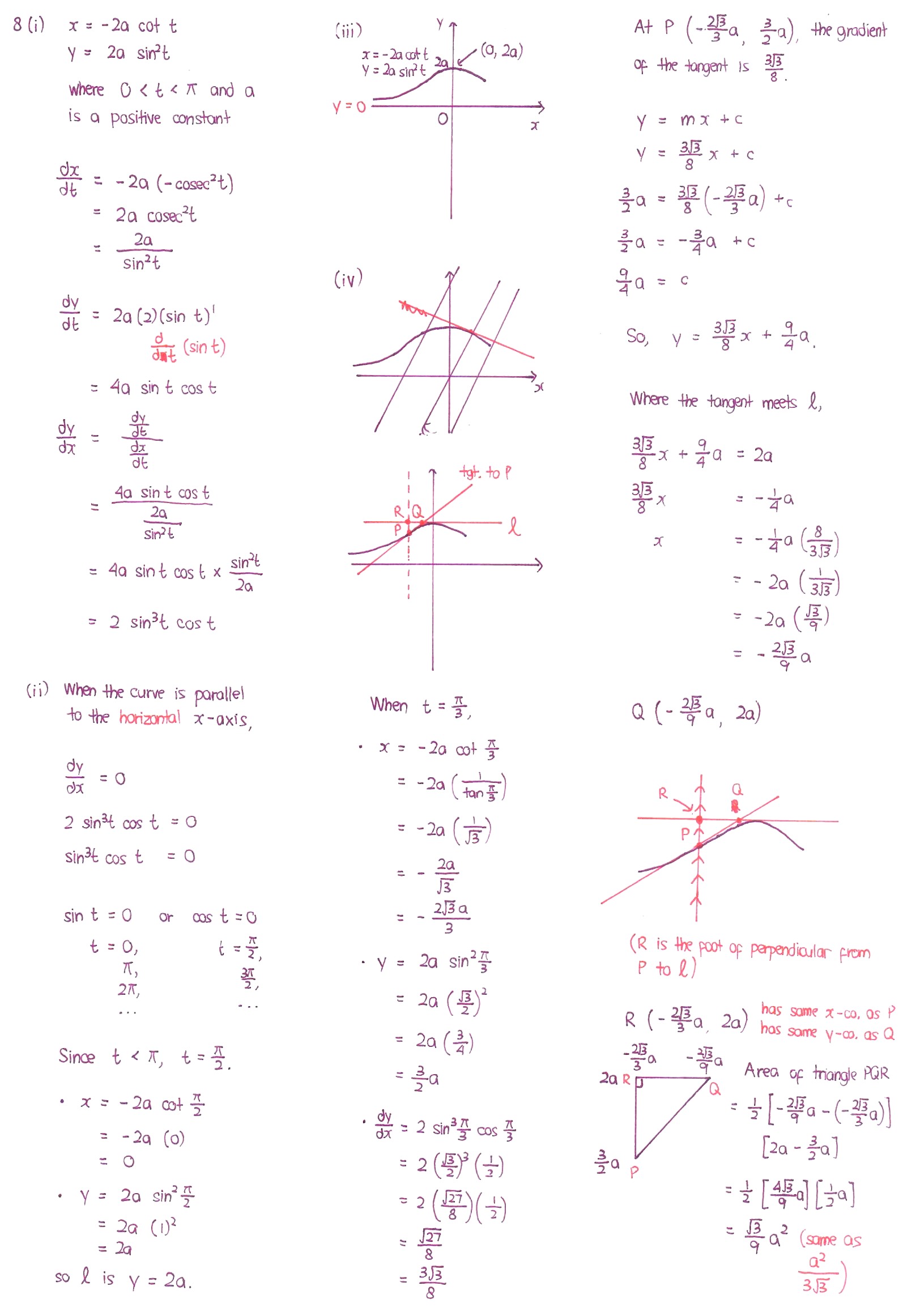
An idea for Q8, which is quite messy. Let me know if you do not understand what I wrote, need more explanation, my workings are too messy etc.
Date Posted:
4 years ago
may i know how u managed to get the graph for 8 ( iii) ?
I plotted this on Desmos parametric calculator using the coordinates (-2a cot t, 2a sin^2 t) and played around with the values of a from 0 to 10.
360 Tutoring Program