Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.

Question
junior college 1 | H2 Maths
One Answer Below
Anyone can contribute an answer, even non-tutors.

would appreciate help!! :)
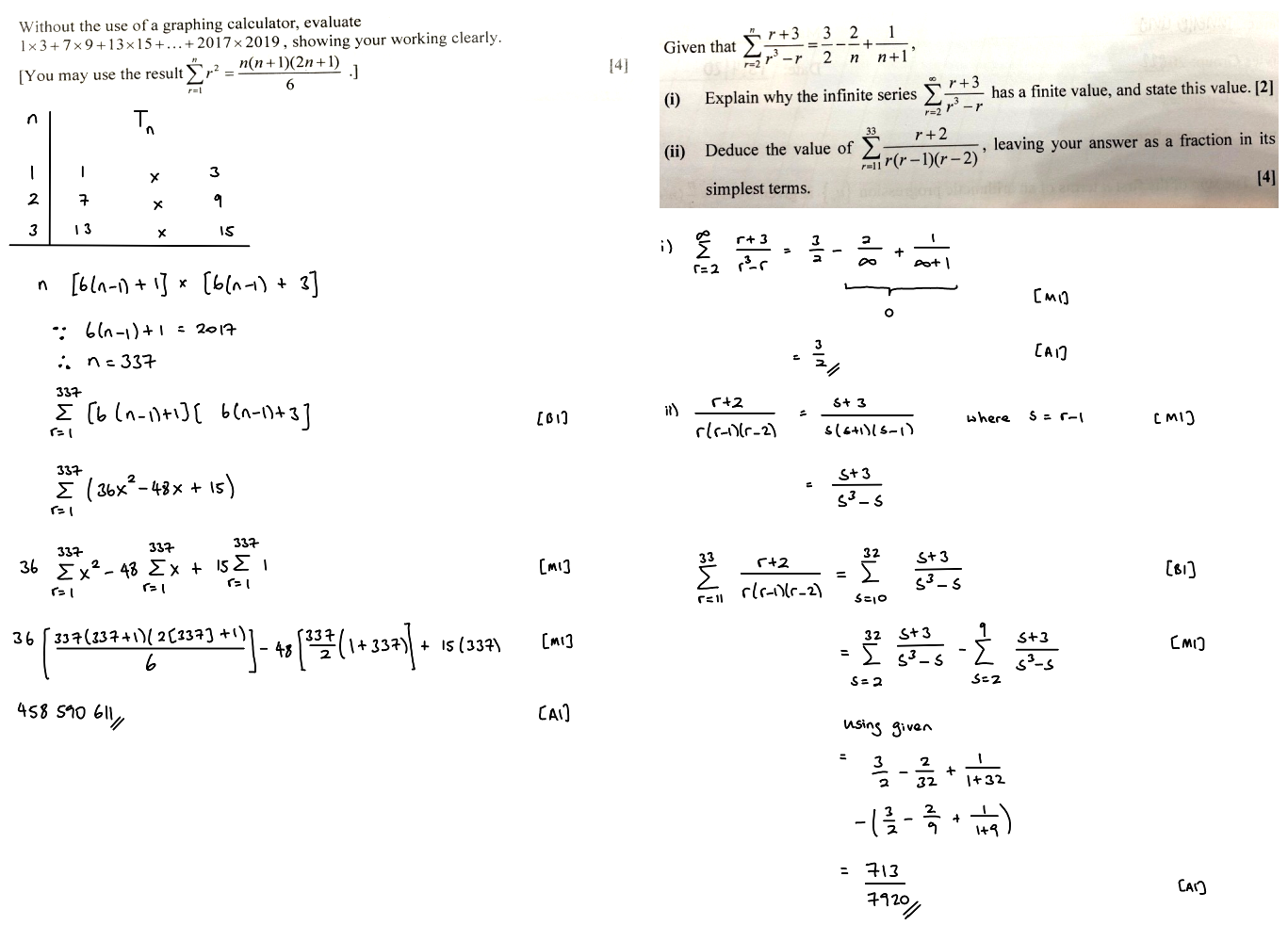
= (1 + 6(1 - 1)) x (1 + 6(1 - 1) + 2)
+ (1 + 6(2 - 1)) x (1 + 6(2 - 1) + 2)
+ (1 + 6(3 - 1)) x (1 + 6(3 - 1) + 2)
+...
+ (1 + 6(337 - 1)) x (1 + 6(337 - 1) + 2)
Notice that each term is in the form
(1 + 6(r - 1) ) x (1 + 6(r -1) + 2)
= (1 + 6r - 6) x (1 + 6r - 6 + 2)
= (6r - 5) x (6r - 3)
= 36r² - 18r - 30r + 15
= 36r² - 48r + 15
So this is simply the sum of
36r² - 48r + 15 to 337 terms.
i.e (first term r = 1, last term 337)
∑ (36r² - 48r + 15)
(Edited)
(Use the formula ½n(n+1) for the term in r)
Notice that as n → ∞ (tends to infinity) ,
2/n → 0
(The numerator is getting bigger and so 2 divided by it will get smaller and smaller and nearer to 0)
Same for 1/(n + 1) since if n → ∞, n+1 also → ∞
This means 3/2 - 2/n + 1/(n + 1) → 3/2
And thus has a finite value of 3/2
(r + 2)/( r(r - 1)(r - 2) ) can be rewritten as
((r - 1) + 3) / [((r - 1) + 1)(r - 1)((r - 1) -1)]
Notice that :
(r + 3)/(r³ - r)
= (r + 3)/(r(r² - 1))
= (r + 3)/(r(r + 1)(r - 1))
= (r + 3)/( (r+1)r(r - 1) )
So if the sum of (r+3)/(r³ - r) is 3/2 - 2/n + 1/(n + 1),
then the sum of (r+2)/(r(r - 1)(r - 2))
= 3/2 - 2/(n - 1) + 1/( (n - 1) + 1)
= 3/2 - 2/(n - 1) + 1/n
Therefore,
To find (first term 11,last term 33) ∑(r+2)/(r(r - 1)(r - 2)) ,
Just find S33 - S10
= 3/2 - 2/(33 - 1) + 1/33
- (3/2 - 2/(10 - 1) + 1/10)
= 3/2 - 2/32 + 1/33 - 3/2 + 2/9 - 1/10
= -1/16 + 1/33 + 2/9 - 1/10
= 713/7920
(first term 11,last term 33) ∑(r+2)/(r(r - 1)(r - 2)) can rewritten as
(first term 10,last term 32) ∑(r+3)/(r³ - r)
since we are effectively starting the summation from 1 term before.
i.e
First term of (r+2)/(r(r - 1)(r - 2)) is when r = 11
= (11 + 2)/(11(11 - 1)(11 - 2))
= 13/(11 x 10 x 9)
This is the same as (r+3)/(r³ - r) when the first term has r = 10
= (10 + 3)(10³ - 10)
= 13/(10(10² - 1)
= 13/(10(10+1)(10-1))
= 13/(10 x 11 x 9)
So,
first term 11,last term 33) ∑(r+2)/(r(r - 1)(r - 2))
= (first term 10,last term 32) ∑(r+3)/(r³ - r)
= S32 - S9
= 3/2 - 2/32 + 1/(32 + 1)
- (3/2 - 2/9 + 1/(9 + 1) )
= 3/2 - 1/16 + 1/33 - 3/2 + 2/9 - 1/10
= 713/7920
Because if it starts with r = 1,
The first term would be
(1+3)(1³ - 1)
= 4/0
And that is undefined since dividing by 0 is undefined
if you sum to just the first term, i.e S1, where r = 2,
S1 = ∑(r+3)/(r³ - r) = (2 + 3)/(2³ - 2)
= 5/6
Using the equivalent formula,
S1 = 3/2 - 2/2 + 1/(2 +1)
= 1/2 + 1/3
= 3/6 + 2/6
= 5/6
The result is the same for both.
Likewise for S2, using r = 3,
S2 = (2 + 3)/(2³ - 2) + (3 + 3)/(3³ - 3)
= 5/6 + 6/24
= 10/12 + 3/12
= 13/12
Using the equivalent formula,
S2 = 3/2 - 2/3 + 1/(3 +1)
= 18/12 - 8/12 + 1/4
= 18/12 - 8/12 + 3/12
= 13/12
The result is also the same. This proves the equivalency for any last term n (where r = n)
(It should be S32 - S9 instead of S33 - S10)
Nevertheless, the end result is still correct as the first term starts from r = 2 and last term is r = 33 for S32. So we still use n = 33 for the sum.
Likewise for S9, the first term starts with r = 2 and last term is r = 10. So we still use n = 10 for the sum.
The same goes for S32 - S9 in the alternative answer . Just change to S31- S8.
The working is still correct since for S31, it is from r = 2 to = 32 and for S8, it is from r = 2 to r = 9.
See 1 Answer