Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.

Question
junior college 1 | H2 Maths
One Answer Below
Anyone can contribute an answer, even non-tutors.

Could I get some help for this question? Thank you !!
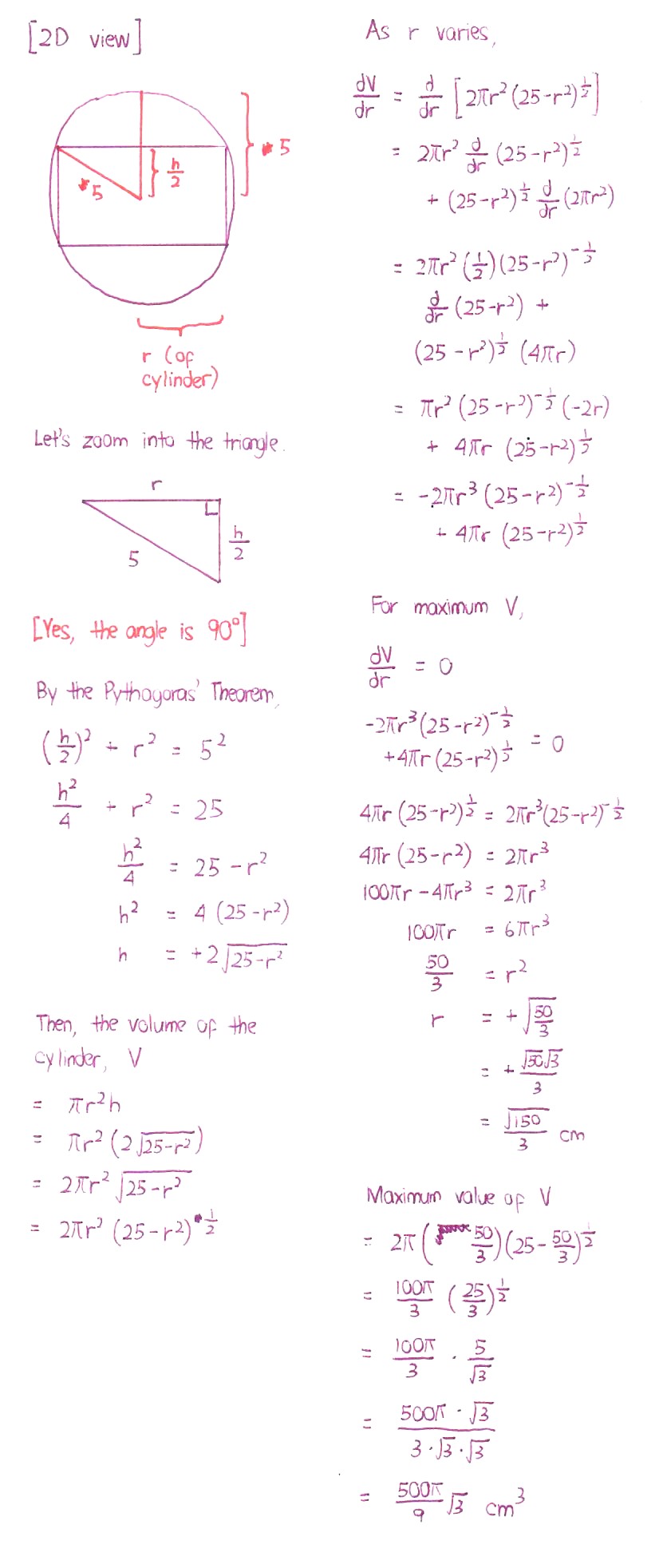
h² + 4r² = 100
h = 2√(25 - r²) *
V = πr² x h = πr² x 2√(25 - r²)
= 2πr²√(25 - r²)
*corrected for, thanks to Eric
V = 2πr²√(25 - r²)
dV/dr = 4πr√(25-r²) + πr²/√(25-r²) x (-2r)
dV/dr
= 4πr√(25 - r²) - 2πr³/√(25 - r²)
set dV/dr = 0,
4πr√(25 - r²) = 2πr³/√(25 - r²)
2r(25 - r²) = r³
3r³ - 50r = 0
r = 0 (rejected) or r² = 50/3
r = 5√(2/3) cm
or -5√(2/3) (rejected)
max V
= 2π x 50/3 x √(25 - 50/3)
= 302.3 cm³
.
.
.
h2 + 4r2 = 100
translates to
h2 = 100 - 4r2
and
h = sqrt (100 - 4r2)
= sqrt (4) * sqrt (25 - r2)
= 2 * sqrt (25 - r2)
and not 2 * sqrt * (25 - 4r2).
See 1 Answer