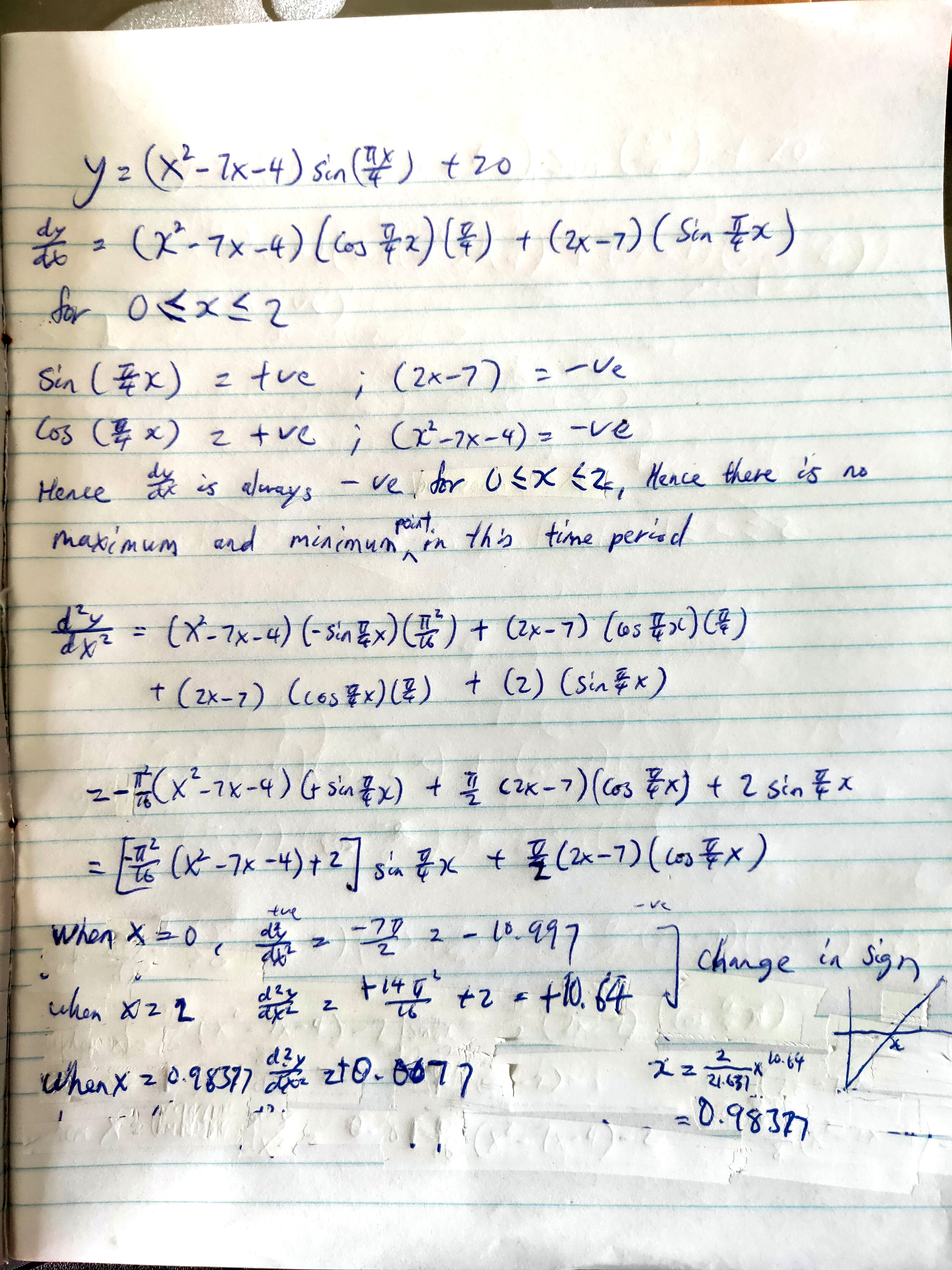Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.
Question
junior college 1 | H2 Maths
One Answer Below
Anyone can contribute an answer, even non-tutors.
Having trouble with this question.
For part (a),
When they mean maximum and minimum, it implies that we have to set dy/dx = 0 right?
I cant seem to solve dy/dx = 0..
Afterwards, we use the 1st Derivative Test to check whether the value is a max or min?
For part (b),
Im assuming we have to use d2y/dx2 = 0? As we are looking at the rate of the decrease and it is neither speeding up nor slowing down.
Thank you
Date Posted: 5 years ago
Views: 387
Should be yes. A constant decrease in the population means a fixed change in its derivative dy/dx, so ITS derivative d2y/dx2, which measures the gradient of dy/dx, must be zero.
Yes. If the decrease is neither slowing down or speeding up, the decrease is constant. I.e dy/dx = constant.
Since dy/dx is a constant, then d²y/dx² = 0
Since dy/dx is a constant, then d²y/dx² = 0
Even though your intuition is correct here, I don't think this question is solvable without the use of a Graphics Display Calculator, which should be allowed at the H2 level. Simply sketch the graph given as 'y' and find maxima and minima points, or use numSolve if your calculator has that feature. However, do let me know if you are not permitted to use the GDC in your examinations.
See 1 Answer
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
First devivative test
Interpolation
Interpolation
Date Posted:
5 years ago
360 Tutoring Program