Gradient

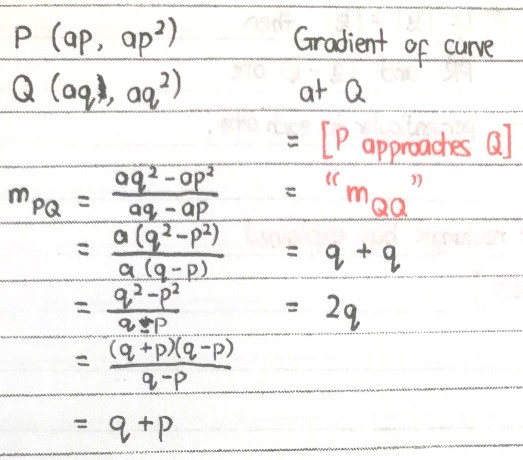
= (y,p - y,q)/(x,p - x,q)

= (ap² - aq²)/(ap - aq)

= a(p + q)(p - q)/(a(p-q))

= p + q


Gradient of line from a chosen point on the curve to any other point on the curve

= (f(c+Δx) − f(c))/Δx


Where the chosen point's x coordinate is c (i.e x = c)

= (f(d) - f(c))/(d - c) , where d = c + ∆x and d is the x coordinate of any other point on the curve



Gradient of PQ

= ( f(aq+(ap-aq)) − f(aq) )/(ap - aq)

= (f(ap) - f(aq))/(ap - aq)

= (ap² - aq²)/(ap-aq)

= p + q

(This is essentially what was found earlier. using point Q, x = aq in this case and your ∆x = ap - aq)



Recalling what you've learned from limits,

Gradient of curve

= limit of (f(c+Δx) − f(c))/Δx when ∆x → 0.

For f(x) when x = c.

(Also known as your dy/dx)


P and Q can be any 2 different points in the circle. They are arbitrary. So we can let Q be our chosen point and P be the other point.


So as your ∆x →0, ap→aq.

I.e the second point is getting closer to and tending towards Q.


Then p → q


So your gradient p + q → 2q

Therefore it's just 2q.

Gradient at point Q is the same as bringing the point P closer to Q (as P comes closer to Q), the chord appears to become closer to an ideal tangent at Q.

So gradient at Q
= Gradient of chord PQ as P —> Q
= “Gradient of QQ” (the ultimate tangent is achieved when P actually reaches Q; just before this, the chord PQ is very close to how a tangent at Q looks like)
= q + q
= 2q

This is how the tangent to a curve is defined to be; the limit when the random point P approaches point Q.

Because this idea involves limits, we do not have to actually be concerned about the unsimplified version of gradient (which contains denominator ap - aq that becomes 0 if p = q).

Unlike conventional mathematics where we cannot allow denominators to become zero at any point in the workings, the limits allow this idea.

Or rather the denominator is eliminated in this case. In other cases with denominator having a (x2 - x1) remaining, this method may not work.

For further reading :

https://brilliant.org/wiki/tangent-line-point/


To convince yourself that it's 2q

y = at²
x = at

so x² = a²t² = a(at²)

y = at² = a²t²/a = x²/a

dy/dx = 2x/a

Sub x = aq,

dy/dx = 2aq/a = 2q