Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.
Question
secondary 3 | A Maths
One Answer Below
Anyone can contribute an answer, even non-tutors.
Pls help me with 13i ASAP thx
Date Posted: 2 years ago
Views: 530
See 1 Answer
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
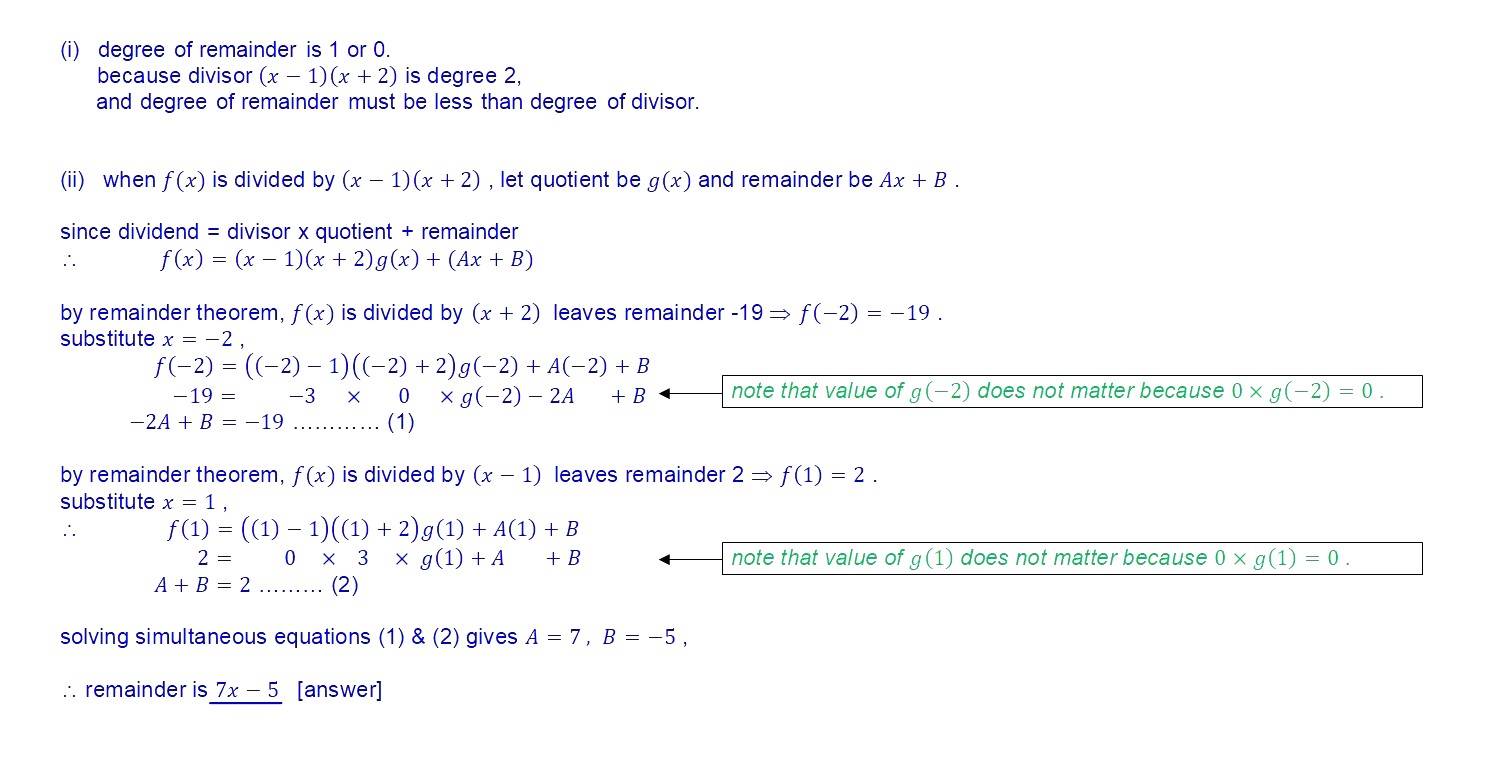
compare with division of integers ...
for example, when any integer is divided by 5, remainder is at most 4.
in general, when any integer is divided by integer n, remainder is at most n-1.
in general, when a polynomial is divided by another polynomial of degree n, remainder is polynomial of degree of at most n-1.
interesting note: degree of f(x) is not even given in this question as it does not affect the solution.
for example, when any integer is divided by 5, remainder is at most 4.
in general, when any integer is divided by integer n, remainder is at most n-1.
in general, when a polynomial is divided by another polynomial of degree n, remainder is polynomial of degree of at most n-1.
interesting note: degree of f(x) is not even given in this question as it does not affect the solution.
Date Posted:
2 years ago
May i know how do u get A=7 and B= -5
You have 2 simultaneous equations with 2 unknowns. Just solve using your preferred method.
Here are possible steps using elimination method ...
-2A + B =-19 ... (1)
A + B = 2 ... (2)
(2) - (1) gives
3A = 21
A = 7
substitute A = 7 into (2), gives
7 + B = 2
B = -5
Here are possible steps using elimination method ...
-2A + B =-19 ... (1)
A + B = 2 ... (2)
(2) - (1) gives
3A = 21
A = 7
substitute A = 7 into (2), gives
7 + B = 2
B = -5
Solving of
- linear equations
- quadratic equations
- simultaneous equations
is very important skill that you should master as you will come across them often in the other maths topics.
- linear equations
- quadratic equations
- simultaneous equations
is very important skill that you should master as you will come across them often in the other maths topics.
Than how to u get the reminder
As explained at the top of the solution, we know that the remainder is at most degree 1 or 0, so we let the remainder be Ax+B, where A & B are constants to be found.
Important is to understand that
dividend = divisor x quotient + remainder.
Compare with division of integers ...
For example, 16 = 3 x 5 + 1
In the same way,
f(x) = (x-1)(x+2) x quotient + Ax+B.
We substitute the given conditions f(-2)=-19 and f(1)=2 to form the 2 equations with 2 unknowns A & B.
By solving the 2 simultaneous equations, we get A = 7 & B =-5.
Since from the beginning our remainder is Ax+B, so answer is remainder is 7x-5.
Important is to understand that
dividend = divisor x quotient + remainder.
Compare with division of integers ...
For example, 16 = 3 x 5 + 1
In the same way,
f(x) = (x-1)(x+2) x quotient + Ax+B.
We substitute the given conditions f(-2)=-19 and f(1)=2 to form the 2 equations with 2 unknowns A & B.
By solving the 2 simultaneous equations, we get A = 7 & B =-5.
Since from the beginning our remainder is Ax+B, so answer is remainder is 7x-5.
360 Tutoring Program