Ask Singapore Homework?
Upload a photo of a Singapore homework and someone will email you the solution for free.
Question
secondary 3 | E Maths
One Answer Below
Anyone can contribute an answer, even non-tutors.
hi, i need help with the coordinates to plot the line stated in part d, tysm!
Date Posted: 3 years ago
Views: 693
See 1 Answer
done
{{ upvoteCount }} Upvotes
clear
{{ downvoteCount * -1 }} Downvotes
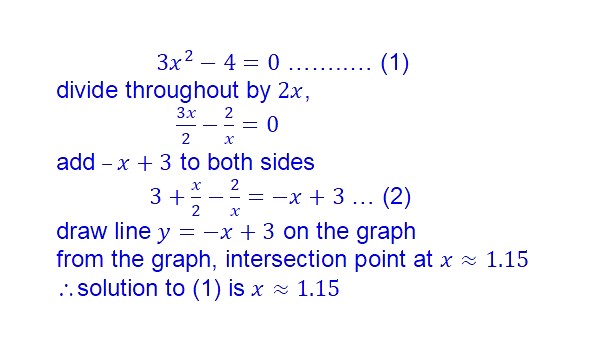
to use an existing curve to solve a new equation (1), you need to rearrange the new equation so that on one side, you have to original curve, as shown in (2).
whatever appears on the right side in (2), is the new line/curve that you need to add to the original curve. where the new line and the original curve intersect, gives the solution to (2), which will also be the solution to (1).
note...
for this question, you are asked to find only the solution where 0<=x<=4. actually, there is another solution at x=-1.15, and if you extend your graph, you will find the the new line & the original curve also intersects at x=-1.15 . you can also solve 3x^2-4=0 with your calculator, to verify that the solutions are indeed + & - 1.15 approximately.
whatever appears on the right side in (2), is the new line/curve that you need to add to the original curve. where the new line and the original curve intersect, gives the solution to (2), which will also be the solution to (1).
note...
for this question, you are asked to find only the solution where 0<=x<=4. actually, there is another solution at x=-1.15, and if you extend your graph, you will find the the new line & the original curve also intersects at x=-1.15 . you can also solve 3x^2-4=0 with your calculator, to verify that the solutions are indeed + & - 1.15 approximately.
Date Posted:
3 years ago
instead of dividing (1) by 2x, you can also ...
divide (1) by 6x, then ...
add -4/(3x)+3 to both sides, ...
giving 3+x/2-2/x = -4/(3x)+3.
adding curve y=-4/(3x)+3 to curve y=3+x/2-2/x will also give you the same solutions as before, but y=-4/(3x)+3 is not a straight line, so this way is even harder work.
generally, for O level E maths, the questions are set such that the additional line that you need is a straight line, so you should aim to get a straight line.
divide (1) by 6x, then ...
add -4/(3x)+3 to both sides, ...
giving 3+x/2-2/x = -4/(3x)+3.
adding curve y=-4/(3x)+3 to curve y=3+x/2-2/x will also give you the same solutions as before, but y=-4/(3x)+3 is not a straight line, so this way is even harder work.
generally, for O level E maths, the questions are set such that the additional line that you need is a straight line, so you should aim to get a straight line.
360 Tutoring Program