y = x²

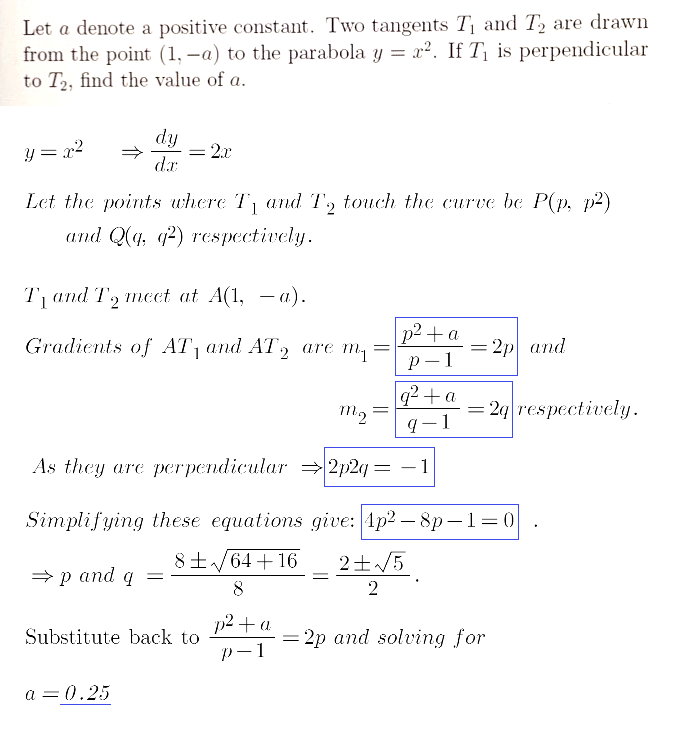
dy/dx = 2x

(take this as the gradient of any tangent to the parabola for some value of x)


Let the coordinates of the tangent point of T1 be (c,c²)

Let the coordinates of the tangent point of T2 be (d,d²)

Gradient of T1 = 2c
Gradient of T2 = 2d


Since the tangents are perpendicular to each other, the product of their gradients is -1.

2c × 2d = -1
4cd = -1
cd = -¼
d = -¼/c = -1/4c ①

But, we can also calculate gradient using the formula (y2 - y1)/(x2 - 1)

gradient of T1 = (c² - (-a)) / (c - 1) = (c² + a)/(c - 1)
gradient of T2 = (d² - (-a)) / (c - 1) = (d² + a)/(d - 1)


Compare the gradients for T1,
(c² + a)/(c - 1) = 2c
c² + a = 2c² - 2c
c² - 2c - a = 0
a = c² - 2c

Likewise for T2,
a = d² - 2d


So, c² - 2c = d² - 2d

Sub ①,

c² - 2c = (-1/4c)² - 2(-1/4c)
c² - 2c = 1 / 16c² + 1 / 2c

Multiply by 16c² on both sides,

16c⁴ - 32c³ = 1 + 8c
16c⁴ - 32c³ - 8c - 1 = 0

Manually factorise,

(4c² + 1)(4c² - 8c - 1) = 0

4c² + 1 = 0
c² = -¼
c = ±0.5i
(Non-real/complex solution)


OR

4c² - 8c - 1 = 0
4c² - 8c = 1
c² - 2c = ¼

So a = ¼

Fun fact : If you actually went on to solve for c and d, you'll get an ordered pair of 1 + ½√5 and 1 - ½√5.

1 + ½√5 is actually the golden ratio (Φ) ≈ 1.618

Thank U all (: